準同型定理
表示
抽象代数学における準同型定理(じゅんどうけいていり、英: fundamental theorem on homomorphisms; 準同型の基本定理, fundamental homomorphism theorem)は、与えられた構造をもつ二つの対象の間の準同型が与えられたとき、その準同型の核と像とを関係づける。
準同型定理は同型定理の証明に利用できる。
以下、群の場合に定理の主張を述べるが、同様の主張はモノイド、ベクトル空間、加群、環などについても成立する。
定理の主張
[編集]- 定理 (群に関する準同型定理)
- 群 G, H および群準同型 f: G → H が与えられたとき、G の正規部分群 K および自然な射影 φ: G → G/K(G/K は剰余群)に対し、K ⊂ ker(f)(f の核)が成り立つならば、群準同型 h: G/K → H が存在して f = h ∘ φ とできる。
この状況を以下の可換図式
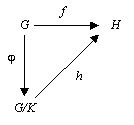
で表すことができる。これはすなわち自然な射影 φ が K を単位元に写す G 上の準同型の中でもっとも一般のものであることを言っている。
定理において K = ker(f) と置けばただちに第一同型定理が得られる。
関連項目
[編集]参考文献
[編集]- Beachy, John A. (1999), “Theorem 1.2.7 (The fundamental homomorphism theorem)”, Introductory Lectures on Rings and Modules, London Mathematical Society Student Texts, 47, Cambridge University Press, p. 27, ISBN 9780521644075.
- Grove, Larry C. (2012), “Theorem 1.11 (The Fundamental Homomorphism Theorem)”, Algebra, Dover Books on Mathematics, Courier Corporation, p. 11, ISBN 9780486142135.
- Jacobson, Nathan (2012), “Fundamental theorem on homomorphisms of Ω-algebras”, Basic Algebra II, Dover Books on Mathematics (2nd ed.), Courier Corporation, p. 62, ISBN 9780486135212.
- Rose, John S. (1994), “3.24 Fundamental theorem on homomorphisms”, A course on Group Theory [reprint of the 1978 original], Dover Publications, Inc., New York, pp. 44–45, ISBN 0-486-68194-7, MR1298629.
