ラミネーション (位相幾何学)
数学の一分野である位相幾何学において、ラミネーション（英: lamination; 葉理、葉紋）とは
曲面のラミネーションは、その閉部分集合の、滑らかな曲線への分割である。
ラミネーションを葉層にすることが可能である場合もある[2]。
例
[編集]- 2次元双曲多様体の測地的ラミネーション（geodesic lamination）は、ある閉部分集合と、測地線によるその集合の葉層からなるものである[3]。それらは写像類群の元のニールセン・サーストン分類や、地震写像の理論で用いられる。
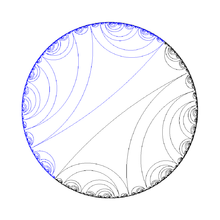
- 2次ラミネーションは、倍角写像の下で不変なものである[4]。このようなラミネーションは 2次写像と関連している[5][6]。これは単位円板内のコードの閉じた集まりである[7]。また、マンデルブロ集合あるいはジュリア集合の位相幾何学的なモデルでもある。
関連項目
[編集]注釈
[編集]- ^ Lamination in The Online Encyclopaedia of Mathematics 2002 Springer-Verlag Berlin Heidelberg New York
- ^ “アーカイブされたコピー”. 2009年7月13日時点のオリジナルよりアーカイブ。2009年6月19日閲覧。 Oak Ridge National Laboratory
- ^ Laminations and foliations in dynamics, geometry and topology: proceedings of the conference on laminations and foliations in dynamics, geometry and topology, May 18-24, 1998, SUNY at Stony Brook
- ^ Houghton, Jeffrey. "Useful Tools in the Study of Laminations" Paper presented at the annual meeting of the The Mathematical Association of America MathFest, Omni William Penn, Pittsburgh, PA, Aug 05, 2010
- ^ Tomoki KAWAHIRA: Topology of Lyubich-Minsky's laminations for quadratic maps: deformation and rigidity (3 heures)
- ^ Topological models for some quadratic rational maps by Vladlen Timorin
- ^ Modeling Julia Sets with Laminations: An Alternative Definition by Debra Mimbs Archived 2011年7月7日, at the Wayback Machine.
参考文献
[編集] | この項目は、位相幾何学に関連した書きかけの項目です。この項目を加筆・訂正などしてくださる協力者を求めています（プロジェクト:数学／Portal:数学）。 |